1.二分查找的前提

• 只有单调的序列才能进行二分查找；

• 一般为单调不减，单调不增需要像 sort() 一样修改比较函数；

2.binary_search( ) 函数

• binary_search( ) 是算法库（algorithm）函数里面的，用于在一个已经排好序的序列（数组或者容器）中查找目标值target；

• 底层实现为进行二分查找，函数返回一个 bool 值来表示目标值 target 是否存在于此序列中

#include <iostream>
#include <vector>
#include <algorithm>
using namespace std;

int main() {
    vector<int> a = {1,2,4,7,8,9,12};
    int target = 8;
    bool retult = binary_search(a.begin(),a.end(),target);	//前两个参数为查找范围始终点，第三个参数为target；
    if(retult)	cout << "yes";
    else	cout << "no";
    return 0;
}

#include <iostream>
#include <algorithm>
using namespace std;

int main() {
    int a[10] = {1,2,4,7,8,9,12};
    int target = 8;
    bool retult = binary_search(a,a+7,target);	//迭代器可以，数组地址也可以
    if(retult)	cout << "yes";
    else	cout << "no";
    return 0;
}

3.lower_bound( ) 和 upper_bound( )函数

1）lower_bound( )

• 是在非降序序列中寻找 target 值的所在地址；

• lower_bound(a,b,target)会返回此序列 [a,b) 中第一个大于等于 target 值的地址；

• 想获得其下标需要使用其返回值减去a的地址，即 a.begin() 或者 a ；

#include <iostream>
#include <algorithm>
using namespace std;

int main() {
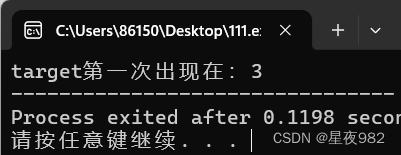
    int a[10] = {1,3,2,11,8,9,10};
    int target = 8;
    sort(a,a+7);
    cout << "target第一次出现在：" << lower_bound(a,a+7,target)-a;
    return 0;
}

#include <iostream>
#include <vector>
#include <algorithm>
using namespace std;

int main() {
    vector<int> a = {1,3,2,11,8,9,10};
    int target = 8;
    sort(a.begin(),a.end());
    cout << "target第一次出现在：" << lower_bound(a.begin(),a.end(),target)-a.begin();
    return 0;
}

2）upper_bound( )

• 与 lower_bound( ) 相同，都是在非降序序列；

• upper_bound(a,b,target) 返回此序列 [a,b) 中第一个大于 target 值的地址；

#include <iostream>
#include <vector>
#include <algorithm>
using namespace std;

int main() {
    vector<int> a = {1,3,2,11,8,8,8,8,8,9,10};
    int target = 8;
    sort(a.begin(),a.end());
    cout << upper_bound(a.begin(),a.end(),target)-a.begin();
    return 0;
}

3）总结

• 当一个序列中存在多个 target 值时，lower_bound( ) 所得到的是序列中第一个 target 值所在的下标a，upper_bound( ) 所得到的是序列中第一个大于 target 值所在的下标b，故此此序列下标 [a,b) 的所有数据均为 target ；