AcWing算法基础课 贪心算法模板题笔记
贪心得到的答案 >= 最优解
贪心得到的答案 <= 最优解
局部最优 -> 全局最优
文章目录
- 1 区间问题
- 例1:区间选点
- 例2:最大不相交区间数量
- 例3:区间分组
- 例4:区间覆盖
- 2 Huffman树
- 例:合并果子
- 3 排序不等式
- 例:排队打水
- 4 绝对值不等式
- 例:货仓选址
- 5 推公式
- 例:耍杂技的牛
1 区间问题
排序 + 维护端点
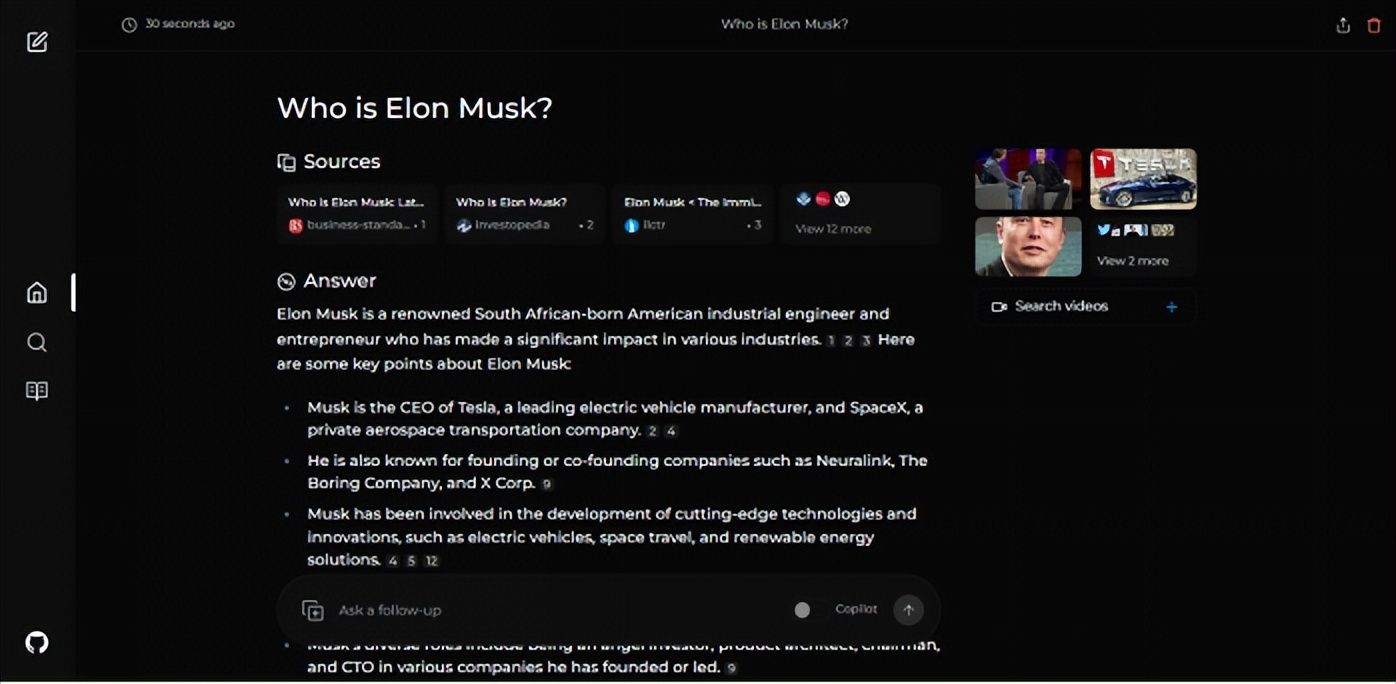
例1:区间选点
AcWing 905. 区间选点
核心思路:维护右端点,判断区间不相交——某区间的左端点大于当前维护区间的右端点
- 将每个区间按右端点从小到大排序
- 从前往后依次枚举每个区间
- 若所枚举区间已包含当前区间的点,则直接pass
- 否则选择当前区间的右端点
理由:若当前区间存在点包含于所枚举区间,则其中必有其右端点,越靠右越有可能被更多的区间包含。
#include <iostream>
#include <cstring>
#include <algorithm>
using namespace std;
typedef pair<int, int> PII;
const int N = 1e5 + 10;
int n;
PII range[N]; // (l, r)
int main() {
scanf("%d", &n);
for (int i = 0; i < n; i++) {
int l, r;
scanf("%d%d", &l, &r);
range[i] = {l, r};
}
sort(range, range + n, [](PII &a, PII &b) {
return a.second < b.second;
});
int res = 0, ed = -2e9;
for (int i = 0; i < n; i++)
if (range[i].first > ed) { // 若不相交(左>当前右)
res++; // 选取当前右端点
ed = range[i].second; // 结束维护,转移至所枚举区间
}
printf("%d\n", res);
return 0;
}
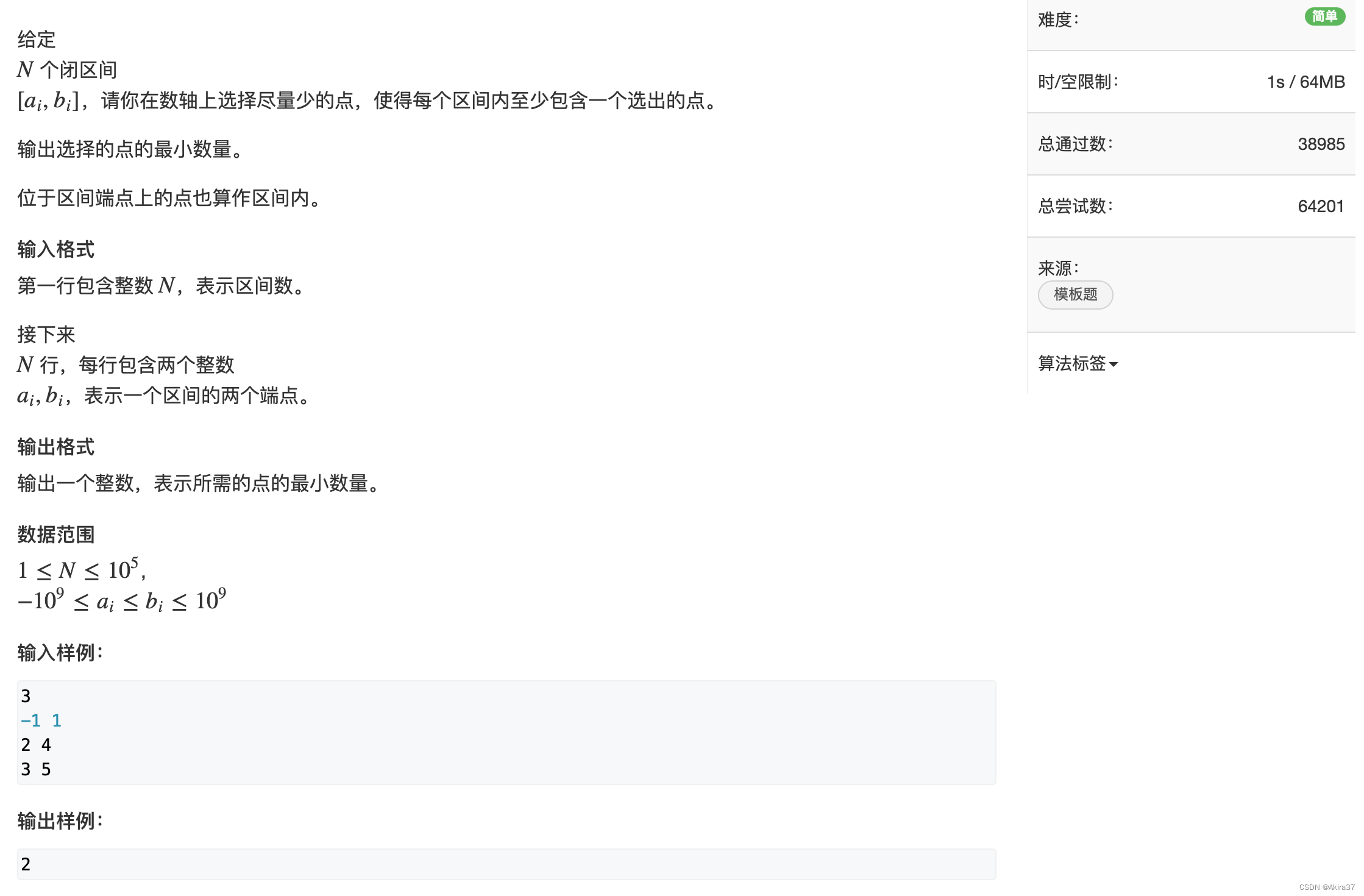
例2:最大不相交区间数量
AcWing 908. 最大不相交区间数量
(核心思路与实际所求的局部最值和上一题完全相同)
- 将每个区间按右端点从小到大排序
- 从前往后依次枚举每个区间
- 若所枚举区间已包含当前区间的点,则直接pass
- 否则选择当前区间的右端点
理由:若当前区间的右端点不包含于所枚举区间,则该两区间必定不相交,故可选择当前区间(实为从当前区间及与其重合的任意区间中任选一)。
/* 同上一例 */
#include <iostream>
#include <cstring>
#include <algorithm>
using namespace std;
typedef pair<int, int> PII;
const int N = 1e5 + 10;
int n;
PII range[N]; // (l, r)
int main() {
scanf("%d", &n);
for (int i = 0; i < n; i++) {
int l, r;
scanf("%d%d", &l, &r);
range[i] = {l, r};
}
sort(range, range + n, [](PII &a, PII &b) {
return a.second < b.second;
});
int res = 0, ed = -2e9;
for (int i = 0; i < n; i++)
if (range[i].first > ed) { // 若不相交(左>当前右)
res++; // 选取当前区间
ed = range[i].second; // 结束维护,转移至所枚举区间
}
printf("%d\n", res);
return 0;
}
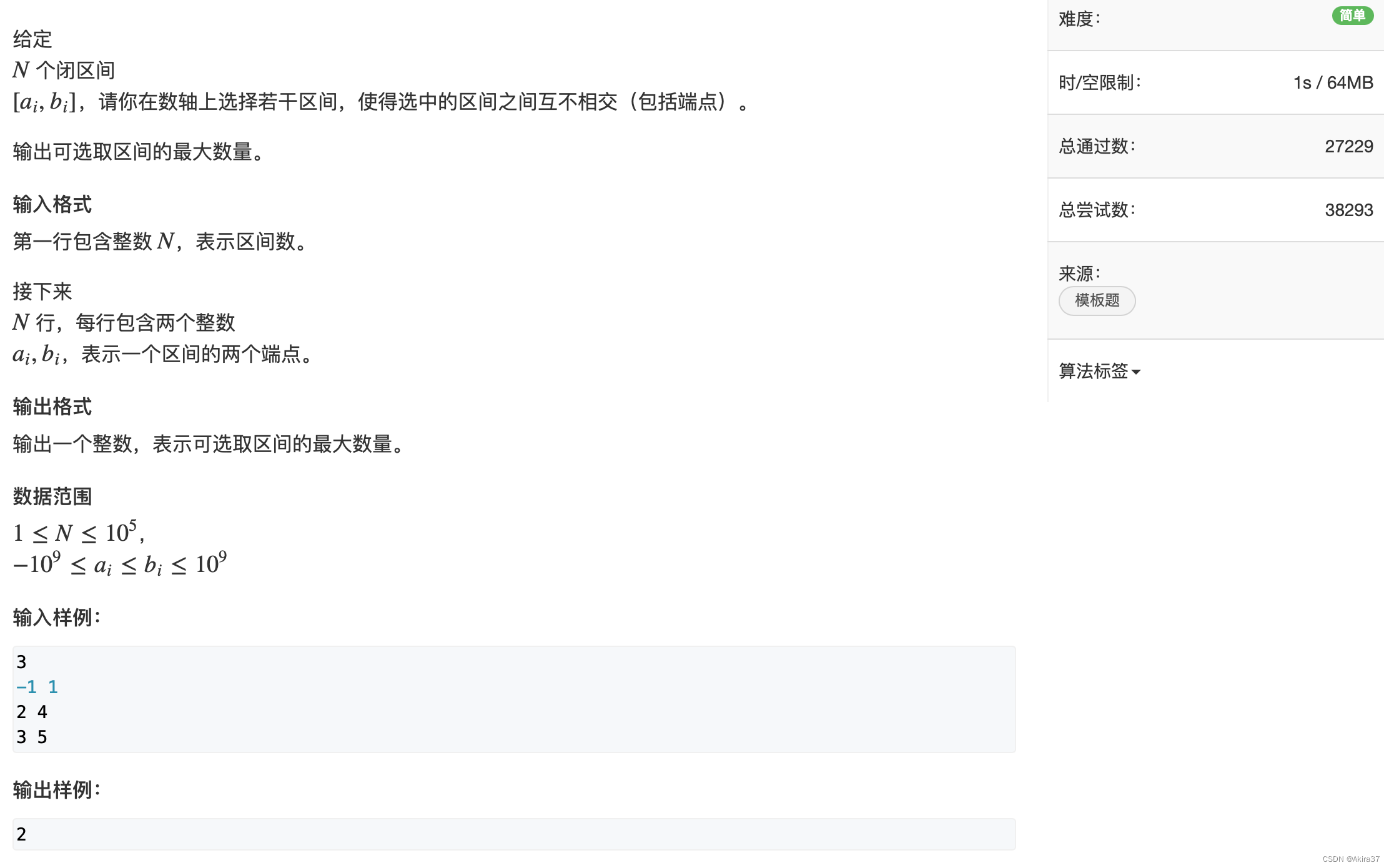
例3:区间分组
AcWing 906. 区间分组
核心思路:同时维护多个右端点,更新其最值
- 将每个区间按左端点从小到大排序
- 从前往后处理每个区间:判断能否将其放到某个现有的组中,即其是否与组内最右区间无交集
- 若不存在这样的组,则开新组放此区间
- 若存在这样的组,则将其放入并更新
可用小根堆快速筛出目前最右区间的右端点最小的组,若满足要求则删除堆顶并将当前区间右端点插入,最终堆中元素个数即为组数。
#include <iostream>
#include <cstring>
#include <algorithm>
#include <queue>
using namespace std;
typedef pair<int, int> PII;
const int N = 1e5 + 10;
int n;
PII range[N]; // (l, r)
int main() {
scanf("%d", &n);
for (int i = 0; i < n; i++) {
int l, r;
scanf("%d%d", &l, &r);
range[i] = {l, r};
}
sort(range, range + n);
priority_queue<int, vector<int>, greater<int> > heap;
for (int i = 0; i < n; i++) {
auto &r = range[i];
if (heap.empty() || heap.top() >= r.first) heap.push(r.second); // 不满足要求则直接插入
else { // 满足要求则删除堆顶后再插入
heap.pop();
heap.push(r.second);
}
}
printf("%d\n", heap.size());
return 0;
}
例4:区间覆盖
AcWing 907. 区间覆盖
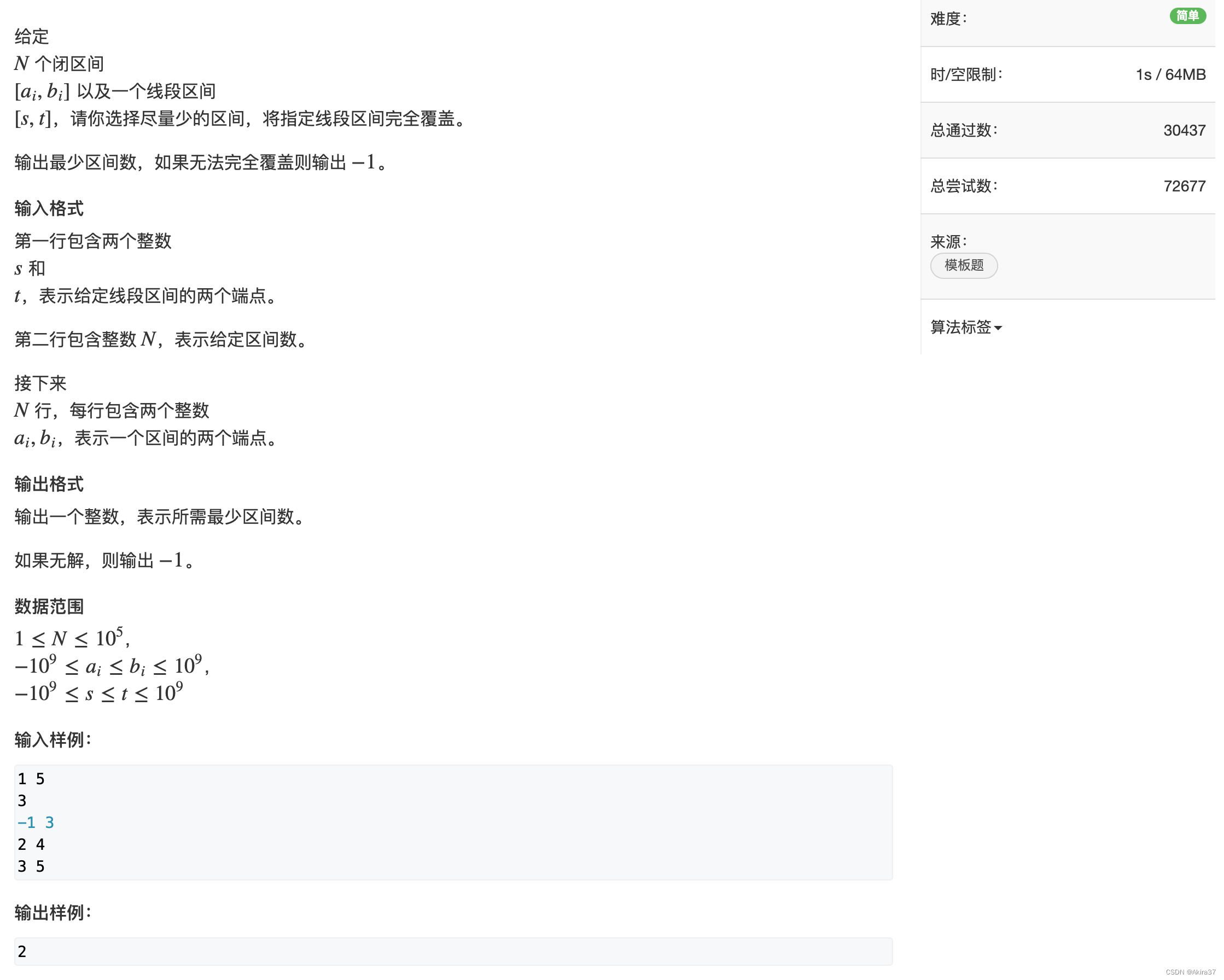
核心思路:覆盖起点,更新起点
- 将每个区间按左端点从小到大排序
- 从前往后依次枚举每个区间,在所有能覆盖
s
s
s 的区间中,选右端点最大的区间(覆盖最多);然后将
s
s
s 更新成右端点的最大值
- 若不存在能覆盖 s s s 的区间,则一定会出现空隙,必无解
理由:选择区间数最少 = 起点 s s s 被赶至终点 t t t 所需次数最少
#include <iostream>
#include <cstring>
#include <algorithm>
using namespace std;
typedef pair<int, int> PII;
const int N = 1e5 + 10;
int n;
PII range[N];
int S, T;
int main() {
scanf("%d%d%d", &S, &T, &n);
for (int i = 0; i < n; i++) {
int l, r;
scanf("%d%d", &l, &r);
range[i] = {l, r};
}
sort(range, range + n);
int res = 0;
bool success = false;
for (int i = 0; i < n;) {
int j = i, max_r = -2e9;
while (j < n && range[j].first <= S) { // 找左端点不超过S的区间的最远右端点
max_r = max(max_r, range[j].second);
j++;
}
if (max_r < S) break; // 最短右端点小于S则说明无法覆盖,必无解
res++;
S = max_r;
if (S >= T) {
success = true;
break;
}
i = j;
}
if (!success) res = -1;
printf("%d\n", res);
return 0;
}
2 Huffman树
带权路径最短
例:合并果子
AcWing 148. 合并果子

核心思想:每次挑出值最小的合并——建立哈夫曼树
#include <iostream>
#include <cstring>
#include <algorithm>
#include <queue>
using namespace std;
int n;
priority_queue<int, vector<int>, greater<int> > heap;
int main() {
scanf("%d", &n);
for (int i = 0; i < n; i++) {
int x;
scanf("%d", &x);
heap.push(x);
}
int res = 0;
while (heap.size() > 1) {
int x1 = heap.top();
heap.pop();
int x2 = heap.top();
heap.pop();
res += x1 + x2;
heap.push(x1 + x2);
}
printf("%d\n", res);
return 0;
}
3 排序不等式
排序
例:排队打水
AcWing 913. 排队打水
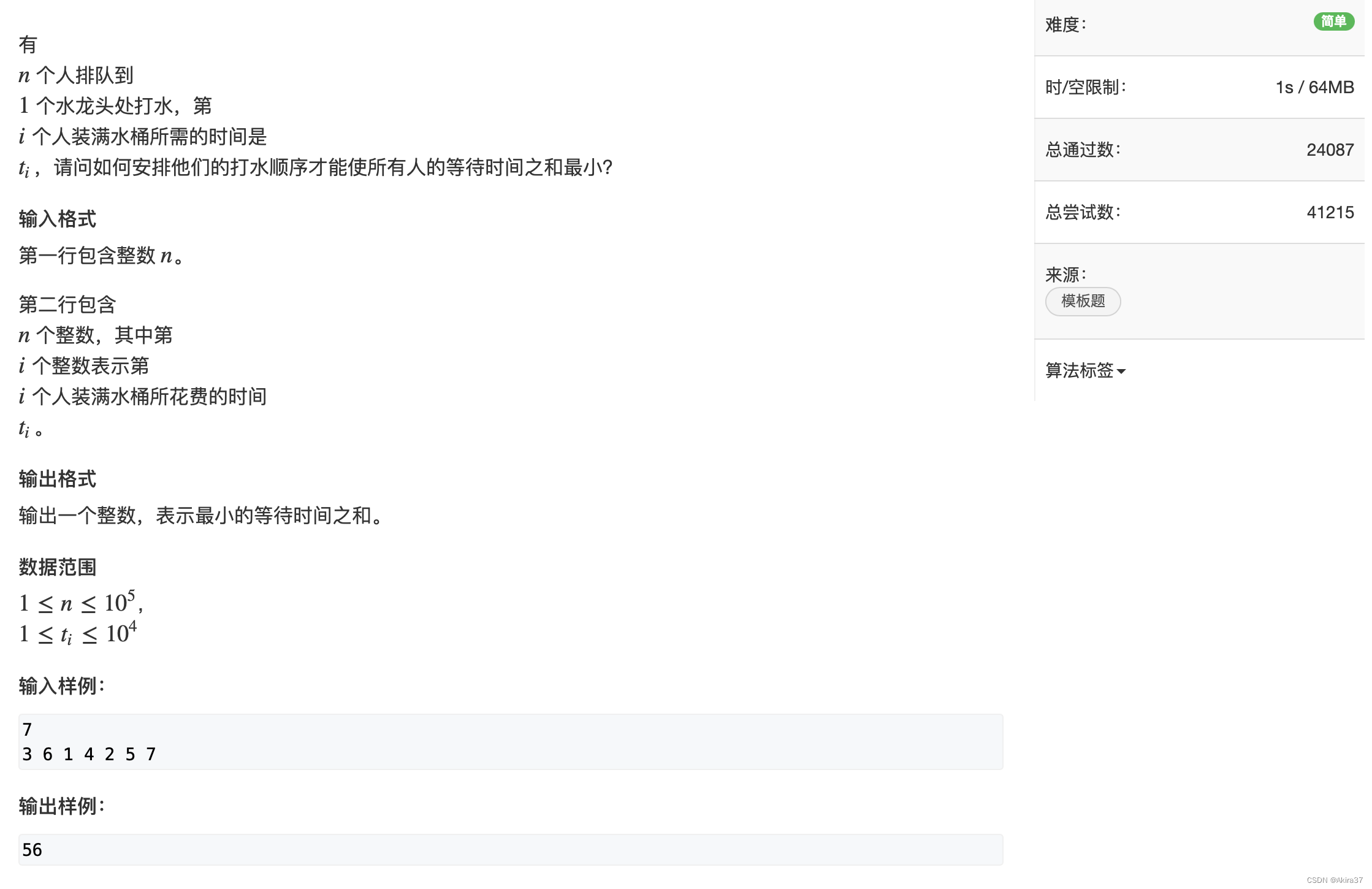
核心思想:将所有数从小到大排序,等待时间之和最小,为 ∑ i = 1 n t i × ( n − i ) \sum_{i=1}^n t_i×(n-i) ∑i=1nti×(n−i) 。
#include <iostream>
#include <cstring>
#include <algorithm>
using namespace std;
const int N = 1e5 + 10;
int n;
int t[N];
int main() {
scanf("%d", &n);
for (int i = 0; i < n; i++) scanf("%d", &t[i]);
sort(t, t + n);
long long res = 0;
for (int i = 0; i < n; i++) res += (long long)t[i] * (n - 1 - i);
printf("%lld\n", res);
return 0;
}
4 绝对值不等式
三角不等式: ∣ ∣ a ∣ − ∣ b ∣ ∣ ≤ ∣ a ± b ∣ ≤ ∣ a ∣ + ∣ b ∣ ||a|-|b||≤|a±b|≤|a|+|b| ∣∣a∣−∣b∣∣≤∣a±b∣≤∣a∣+∣b∣
常见题型:求 f ( x ) = ∣ x 1 − x ∣ + ∣ x 2 − x ∣ + ⋯ + ∣ x n − x ∣ ( x 1 ≤ x 2 ≤ ⋯ ≤ x n ) f(x)=|x_1-x|+|x_2-x|+\cdots+|x_n-x|\ (x_1≤x_2≤\cdots≤x_n) f(x)=∣x1−x∣+∣x2−x∣+⋯+∣xn−x∣ (x1≤x2≤⋯≤xn) 的最值。
例:货仓选址
AcWing 104. 货仓选址
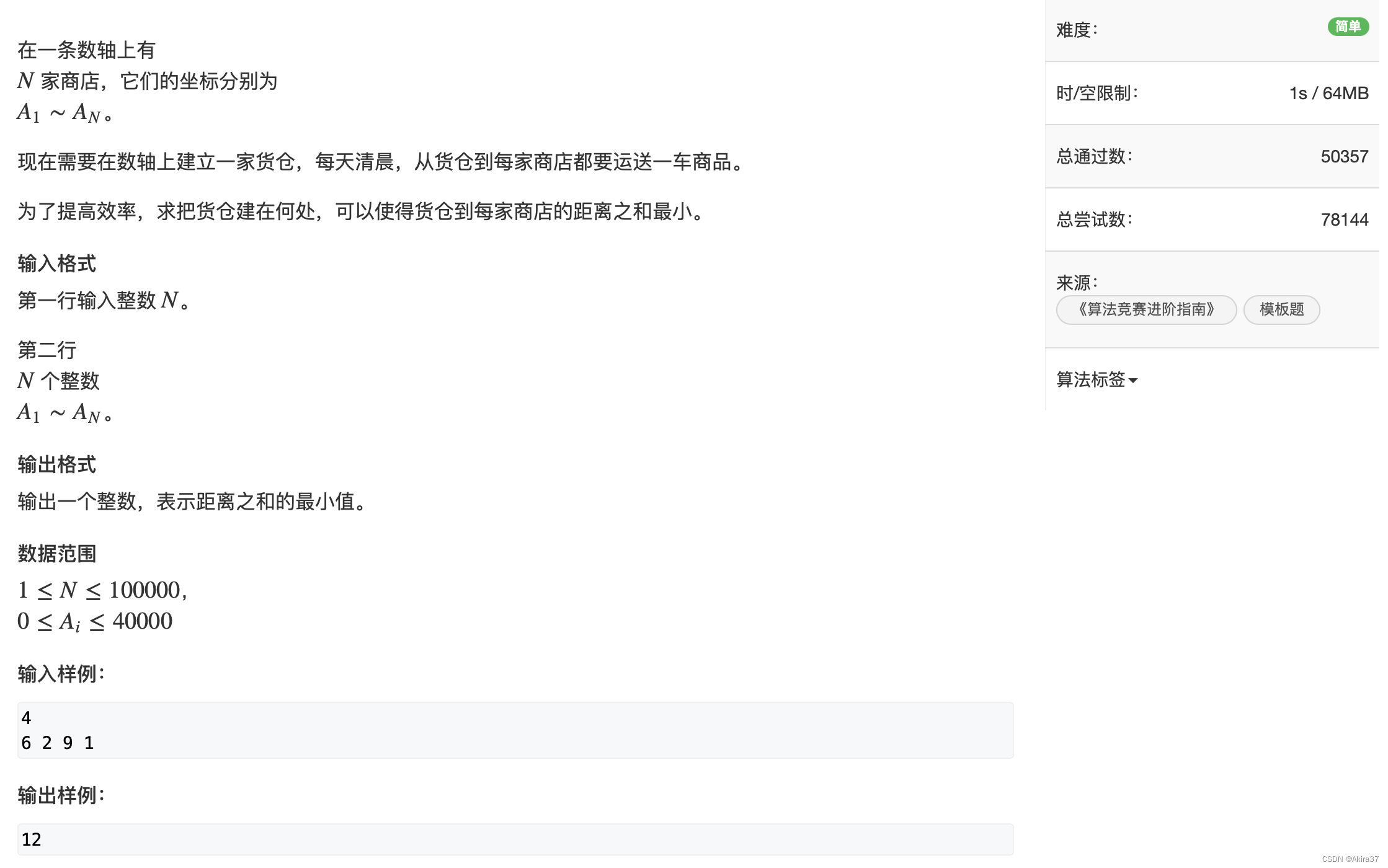
由绝对值三角不等式得 ∣ a − x ∣ + ∣ b − x ∣ ≥ ∣ a − b ∣ |a-x|+|b-x|≥|a-b| ∣a−x∣+∣b−x∣≥∣a−b∣ ,当且仅当 x ∈ [ a , b ] x\in[a,\ b] x∈[a, b] 时取得等号。要求 f ( x ) f(x) f(x) 的最小值,对其两两分组后放缩得 f ( x ) = ( ∣ x 1 − x ∣ + ∣ x n − x ∣ ) + ( ∣ x 2 − x ∣ + ∣ x n − 1 − x ∣ ) + ⋯ ≥ ( x n − x 1 ) + ( x n − 1 − x 2 ) + ⋯ \begin{align*} f(x) &=(|x_1-x|+|x_n-x|)+(|x_2-x|+|x_{n-1}-x|)+\cdots \\ &≥(x_n-x_1)+(x_{n-1}-x_2)+\cdots \end{align*} f(x)=(∣x1−x∣+∣xn−x∣)+(∣x2−x∣+∣xn−1−x∣)+⋯≥(xn−x1)+(xn−1−x2)+⋯ 逐项逼近最终可得: f ( x ) f(x) f(x) 取得最小值时, x x x 为有序数列 x 1 , x 2 , ⋯ , x n x_1,x_2,\cdots,x_n x1,x2,⋯,xn 的中点——当 n n n 为奇数时, x = x n + 1 2 x=x_{\frac{n+1}2} x=x2n+1(即为中位数);当 n n n 为偶数时, x = x n 2 x=x_\frac{n}2 x=x2n 或 x = x n 2 + 1 x=x_{\frac n2+1} x=x2n+1。
#include <iostream>
#include <cstring>
#include <algorithm>
using namespace std;
const int N = 1e5 + 10;
int n;
int a[N];
int main() {
scanf("%d", &n);
for (int i = 0; i < n; i++) scanf("%d", &a[i]);
sort(a, a + n);
int res = 0;
for (int i = 0; i < n; i++) res += abs(a[i] - a[n / 2]);
printf("%d\n", res);
return 0;
}
5 推公式
例:耍杂技的牛
AcWing 125. 耍杂技的牛

思路:越重、越强壮的越应放下面,故按 w i + s i w_i+s_i wi+si 从小到大排序,表示从上到下堆叠。
#include <iostream>
#include <cstring>
#include <algorithm>
using namespace std;
typedef pair<int, int> PII;
const int N = 5e4 + 10;
int n;
PII cow[N]; // (w_i, s_i)
int main() {
scanf("%d", &n);
for (int i = 0; i < n; i++) scanf("%d%d", &cow[i].first, &cow[i].second);
sort(cow, cow + n, [](PII &a, PII &b) {
return a.first + a.second < b.first + b.second;
});
int res = -2e9, sum = 0;
for (int i = 0; i < n; i++) {
res = max(res, sum - cow[i].second);
sum += cow[i].first;
}
printf("%d\n", res);
return 0;
}